Hooke’s Law: Statement, Equation, Graph, Applications, Limitations

What is Hooke’s Law?

Hooke’s law is a principle of engineering mechanics and physics related to the properties of a material. There are basically two statements for Hooke’s law; discovered by the English scientist Robert Hooke. The first statement relates to the elongation of spring subject to the application of force. And the second statement is known as the law of elasticity and relates the relation of stress and strain of materials. In this article, we will explore more details about Hooke’s law.

Hooke’s Law Statement for Springs

For Springs, Hooke’s law states that the force (F) required to extend or compress a spring by some distance (x) varies proportionately with respect to that distance. This means more force will be required to elongate a spring more and vice versa.

When elastic materials like springs are stretched using a force (F), deformation of atoms and molecules occurs until stress is applied and they get back to their initial state when the stress is removed.

Hooke’s Law Equation for Spring

Mathematically, Hooke’s law for Springs is expressed as:

F= -k.x

Where, In the SI unit

• F is the force; N
• x is the extension or compression of the spring; mm
• k is the constant of proportionality also known as the spring constant in N/m

The negative sign in Hooke’s law is explaining the force as a restoring force that is trying to return the spring to its equilibrium position

Let us consider a spring with the load application as shown in the figure.

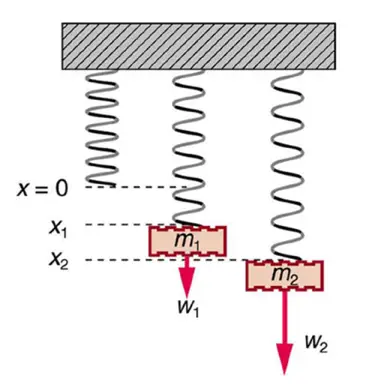
The figure above (Fig. 1) shows the stable condition of the spring when no load is applied to the spring at x=0; the condition of the spring when it gets elongated to the amount x₁ under the load of m₁; and the condition of the spring when it gets elongated to x₂ under the influence of load m₂.

Depending on the material, different springs will have different spring constants.

Hooke’s Law Statement relating Stress & Strain

With respect to the stress and strain of a material, Hooke’s law states that the stress of a material is proportional to its strain within the elastic limit of the material.

The strain will remain in the body as long as the stress acts and when the stress is removed the body will regain its shape. This property of materials is known as elasticity. So basically, Hooke’s law provides the basis for elasticity and so it is known as the elasticity principle or law of elasticity.

Hooke’s law Formula

Mathematically, within the elastic region of a material, Hooke’s law formula is expressed as

σ = Eε

Where, in SI units

• σ is the stress; Pa
• E is the modulus of elasticity or Young’s modulus, Pa (Normally GPa)
• ε is the strain, dimensionless

Hooke’s Law Graph

The below graph (Fig. 2) shows the stress-strain curve for a low-carbon steel material to explain Hooke’s law

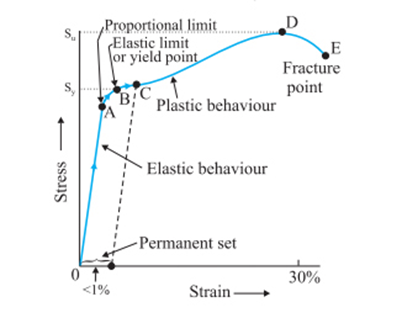
The material tends to have elastic behavior up to the yield strength point (Point B), after that the material loses its elasticity and exhibits plasticity.

From the origin until the proportional limit (Point A) near the yield point (Point B), the straight line implies that the material is following Hooke’s law. But, beyond the elastic limit that is between the proportional limit and yield strength, the material starts losing its elastic nature and exhibits plasticity. The area that is under the curve from the origin to the proportional limit falls under the elastic range. The area that is under the curve from a proportional limit to the rupture point falls under the plastic range. In the plastic region, Hooke’s law is not applicable.

In many real-life situations such as wind blowing on a tall building, and a musician plucking a string of a guitar, the deformation is proportional to the stress/Force and Hooke’s law holds good.

Applications of Hooke’s Law

The applications of Hooke’s Law are as follows:

1. Hooke’s Law is used in all branches of science and engineering; For understanding the behavior of elastic materials there is no substitute for Hooke’s law.
2. It is used as the fundamental principle behind the manometer, the balance wheel of the clock, and a spring scale.
3. Foundation for seismology, molecular mechanics, and acoustics.

Limitations of Hooke’s Law

Even though Hooke’s law is used extensively in Engineering, it’s not a universal principle. The law is not applicable as soon as the elastic limit of a material is exceeded. Normally for solid particles, Hooke’s law provides accurate results when the deformations are small. Many materials deviate from Hooke’s law even well before reaching the elastic limit.

Hooke’s Law calculations

• In Engineering studies, Hooke’s law equation is widely used to calculate the Force required for getting the desired deformation. For example, in spring hanger supports used in piping engineering Hooke’s law is used to know how much pipe weight the spring will carry while allowing desired thermal movement.
• Spring Constant or Young’s modulus is decided following Hooke’s law calculations. For Example, If 2,000 N force is applied to displace a spring by 0.5 m, it’s Spring Constant as per Hooke’s law calculation will be k=F/x=2000/0.5=4000 N/m